ESR - Equivalent series resistance of capacitors
Boovysa, Milano, 2018.

This page is in the "junk" directory! Make sure you check some better material as well, on the main page :-)

Hi folks, Have you ever wondered why people put two capacitors in parallel in many circuits, such as the rectifier in the figure, here?



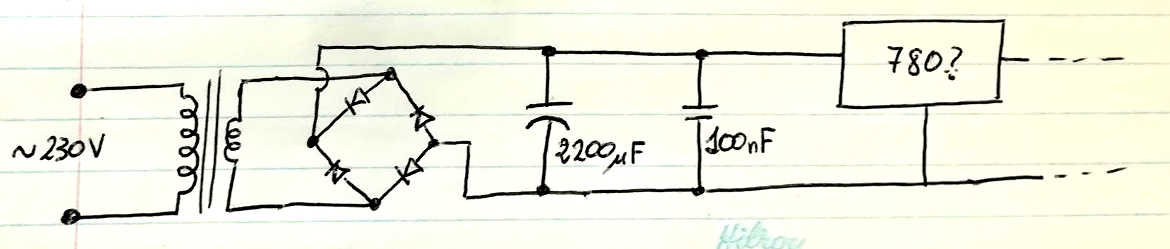
You can see a big capacitor (electrolitic, 2200 uF) and a small one (100 nF, 0.1 uF) in parallel. The explanation circulating on internet sounds like "the big capacitor acts as a reservoir while the small one filters the noise". Or "big and small capacitors have different transfer functions, therefore they have different filtering capabilities".

This is actually misleading. Do you recall the impedance formula for a capacitor (the reactive capacitance Xc)? Xc = -1/(2 pi f C)

This means that if you put two capacitors in parallel, you will see that capacitances simply sum up and you will get the very same type of transfer function, just for a slightly bigger capacitor. In the example, we would just have an equivalent capacity of (2200 uF + 0.1 uF = 2200.1 uF). In other words: nothing changes.
In fact, a big capacitor would in principle act as a low-pass filter and cut out every possible high frequency noise, in a much better way than a small capacitor would do.

The real reason why we should put one big and one small capacitors right next to each other lies in the non-ideal behavior of these devices. In particular, it deals with the fact that every component have an internal resistance, termed ESR (Equivalent Series Resistance). Check out the wikipedia page.

Actually, an even more realistic model of a capacitor also includes a series inductance, although it usually has some effect only at pretty high frequencies:




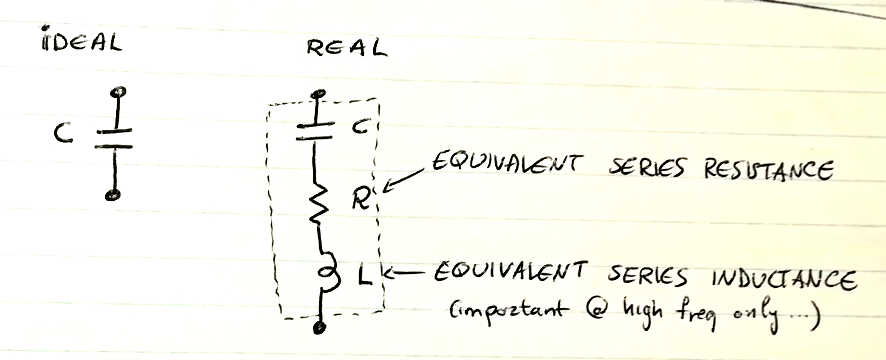
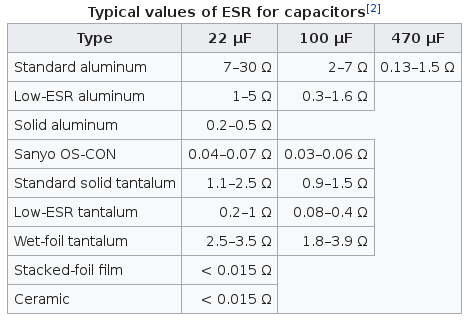
The point is: large capacitors such as electrolytic ones turn out to have a big ESR, while small capacitors (in particular ceramic ones) have a very small one. Check out the following table (freely taken from Wikipedia): electrolitic capacitors can have resistances up to 30 Ohm (!!!) while ceramic ones can go below 0.015 Ohm.



For this reason, in presence of a high frequency noise, a large capacitor could still behave as a 30 Ohm resistor (I'm neglecting the ESL inductance here). In other words, it will not efficiently remove the noise. This is why we should add a small capacitor, maybe of ceramic type.

So, ok, "big capacitors are the reservoir, small capacitors filter the noise" is true... but the reason lies in the non-ideality of the component, not in the amount of capacitance itself.

Check out this nice link: http://www.ecircuitcenter.com/Circuits/cmodel1/cmodel1.htm

Cheers!
-Stefano       -> BACK TO THE HOMEPAGE <-